Research
Interests

- Multivariate splines
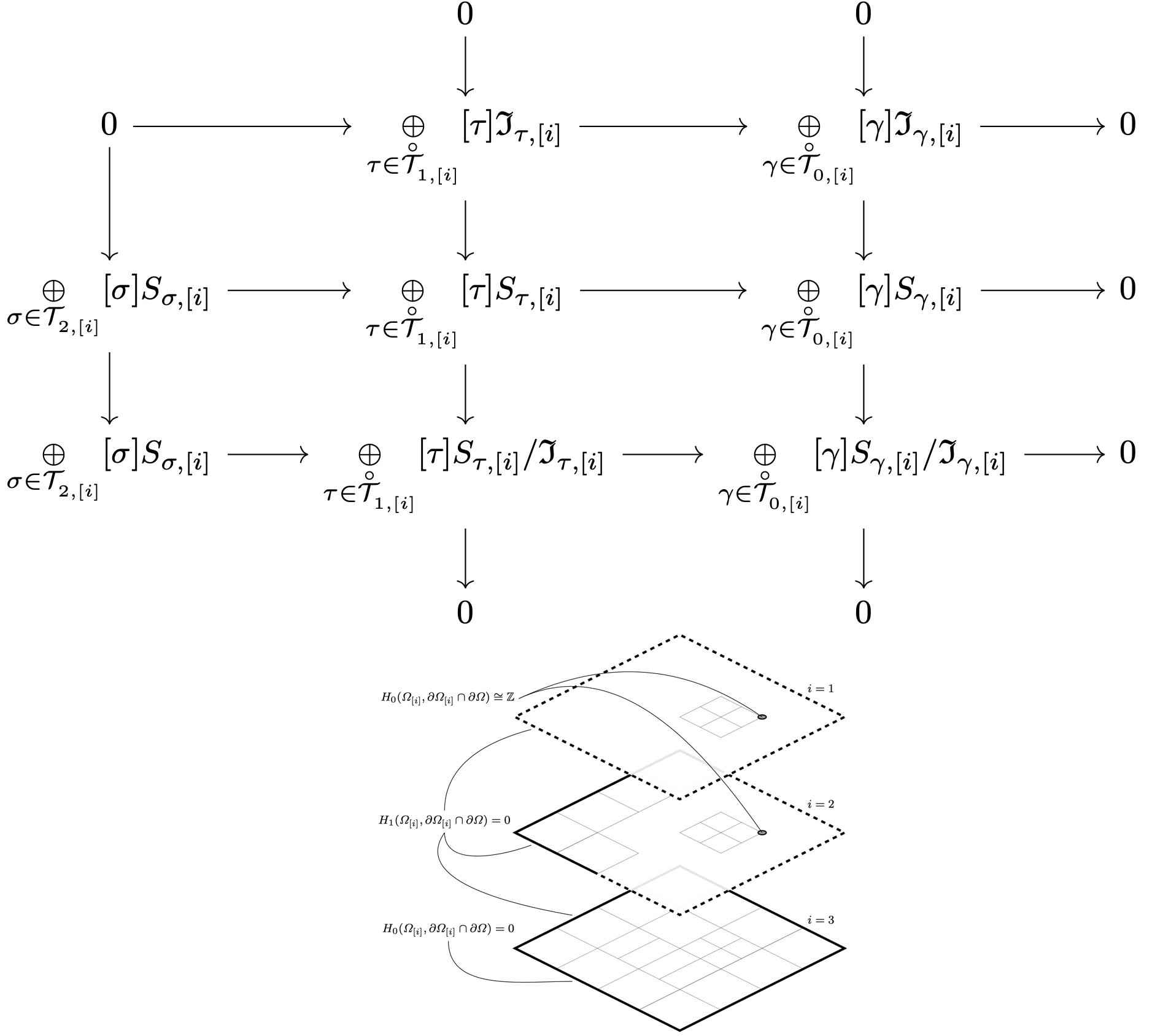
- Isogeometric analysis
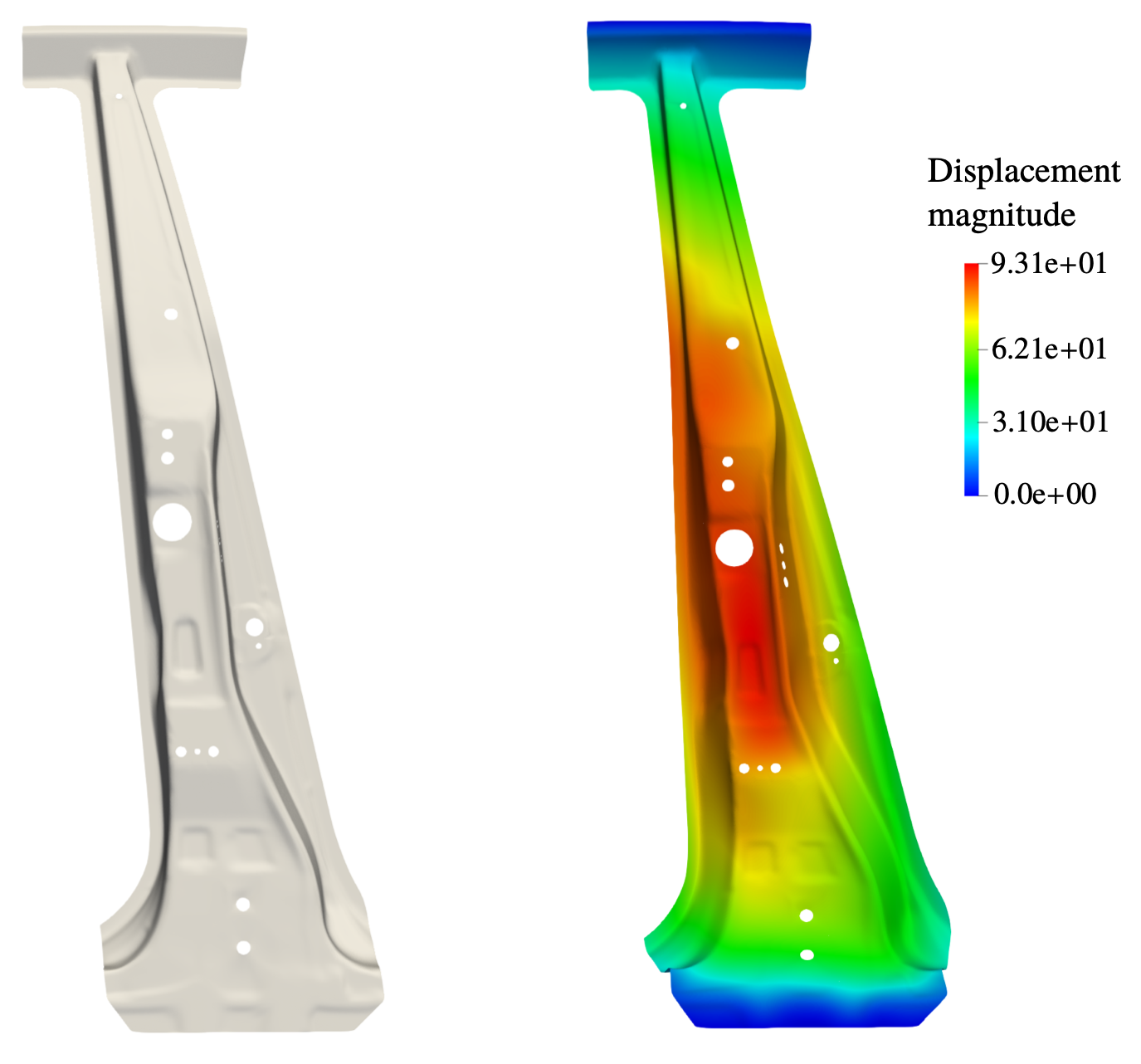
- Computer-aided geometric design
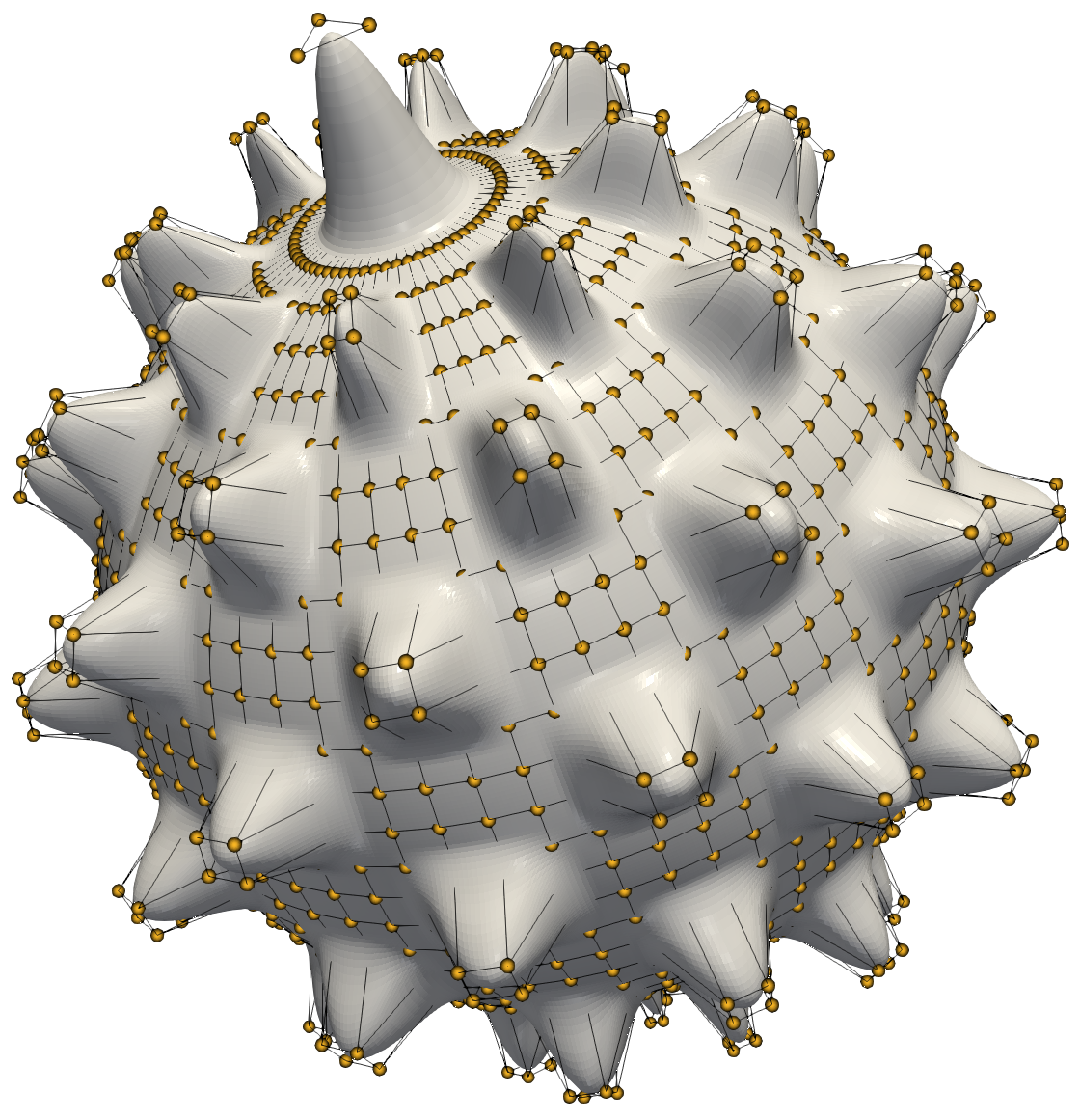
- Structure-preserving discretizations
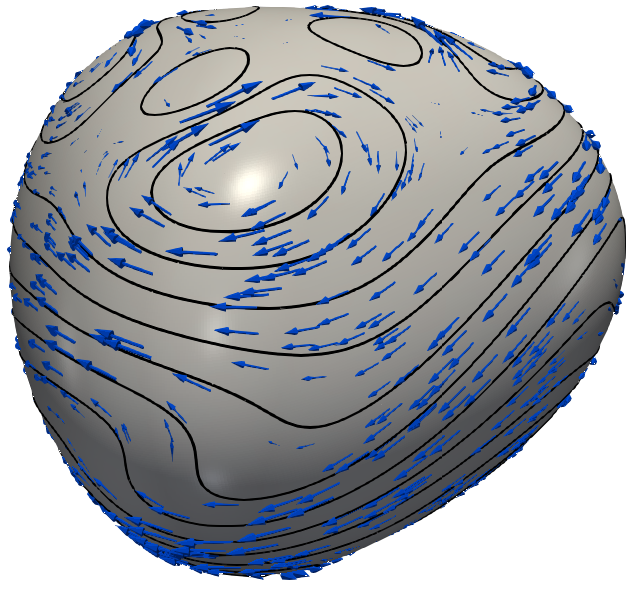
- Scientific machine learning

Thesis options for students
For BSc and MSc thesis projects, consult this list on Kees Vuik’s webpage.
Scientific publications
Preprints
-
Marcro-element refinement schemes for Bezier projection with Truncated Hierarchical B-splines
K. Dijkstra, C. Giannelli and D. Toshniwal. -
Construction of exact refinements for the two-dimensional HB/THB-spline de Rham complex.
D. Cabanas, K. Shepherd, D. Toshniwal and R. Vazquez.
arXiv:2502.19542 (math.NA) -
Isogeometric de Rham complex discretization in solid toroidal domains.
F. Patrizi and D. Toshniwal.
arXiv:2106.10470 (math.NA) -
Decoupled structure-preserving discretization of incompressible MHD equations with general boundary conditions.
Y. Zhang, A. Palha, A. Brugnoli, D. Toshniwal and M. Gerritsma.
arXiv:2410.23973 (math.NA)
Published/Accepted
-
Locally-verifiable sufficient conditions for exactness of the hierarchical B-spline discrete de Rham complex in ℝn.
K. Shepherd and D. Toshniwal.
Foundations of Computational Mathematics, 2024. paper (open access) -
A characterization of linear independence of THB-splines in ℝn and application to B´ezier projection.
K. Dijkstra and D. Toshniwal.
Springer INdAM series: Approximation Theory and Numerical Analysis meet Algebra, Geometry, Topology, 2024. paper -
A comparison of smooth basis constructions for isogeometric analysis.
H.M. Verhelst, P. Weinmüller, A. Mantzaflaris, T. Takacs and D. Toshniwal.
Computer Methods in Applied Mechanics and Engineering, 419, Article 116659, 2024. paper (open access) -
Almost C1 splines: Biquadratic splines on unstructured quadrilateral meshes and their application to fourth order problems.
T. Takacs and D. Toshniwal.
Computer Methods in Applied Mechanics and Engineering, 403 A, Article 115640, 2023. paper (open access) -
Algebraic methods to study the dimension of supersmooth spline spaces.
D. Toshniwal and N. Villamizar.
Advances in Applied Mathematics, 142, Article 102412, 2023. paper (open access) -
An optimally convergent smooth blended B-spline construction for semi-structured quadrilateral and hexahedral meshes.
K.J. Koh, D. Toshniwal and F. Cirak.
Computer Methods in Applied Mechanics and Engineering, 399, Article 115438, 2022. paper, preprint -
Quadratic splines on quad-tri meshes: Construction and an application to simulations on watertight reconstructions of trimmed surfaces.
D. Toshniwal.
Computer Methods in Applied Mechanics and Engineering, 388, Article 114174, 2022. paper (open access) -
Isogeometric discrete differential forms: Non-uniform degrees, Bezier extraction, polar splines and flows on surfaces.
D. Toshniwal and T.J.R. Hughes.
Computer Methods in Applied Mechanics and Engineering, 376, Article 113576, 2021. paper (open access) -
Polynomial spline spaces of non-uniform bi-degree on T- meshes: Combinatorial bounds on the dimension.
D. Toshniwal, B. Mourrain and T.J.R. Hughes.
Advances in Computational Mathematics, 47, Article 16, 2021. paper (open access) -
Counting the dimension of splines of mixed smoothness: A general recipe, and its applications to meshes of arbitrary topologies.
D. Toshniwal and M. DiPasquale.
Advances in Computational Mathematics, 47, Article 6, 2021. paper (open access) -
A general class of C1 smooth rational splines: Applications to construction of exact ellipses and ellipsoids.
H. Speleers and D. Toshniwal.
Computer-Aided Design, 132, Article 102982, 2021. paper (open access) -
The divergence-conforming immersed boundary method: Application to vesicle and capsule dynamics.
H.Casquero, C. Bona-Casas, D. Toshniwal, T.J.R. Hughes, H.Gomez and Y.J.Zhang.
Journal of Computational Physics, 425, Article 109872, 2021. paper (open access) -
Smooth multi-patch discretizations for Isogeometric Analysis.
T.J.R. Hughes, G. Sangalli, T. Takacs and D. Toshniwal.
Handbook of Numerical Analysis, 22, 2021. paper -
Dimension of polynomial splines of mixed smoothness on T-meshes.
D. Toshniwal and N. Villamizar.
Computer Aided Geometric Design, 80, Article 101880, 2020. paper (open access) -
A Tchebycheffian extension of multi-degree B-splines: Algorithmic computation and properties.
R.R. Hiemstra, T.J.R. Hughes, C. Manni, H. Speleers and D. Toshniwal.
SIAM Journal on Numerical Analysis, 58(2), 1138-1163, 2020. paper -
Seamless integration of design and Kirchhoff-Love shell analysis using analysis-suitable unstructured T-splines.
H. Casquero, X. Wei, D. Toshniwal, A. Li, T.J.R. Hughes, J. Kiendl and Y.J. Zhang.
Computer Methods in Applied Mechanics and Engineering, 360, Article 112765, 2020. paper -
Multi-degree B-splines: Algorithmic computation and properties.
D. Toshniwal, H. Speleers, R. Hiemstra, C. Manni and T.J.R. Hughes.
Computer Aided Geometric Design, 76, Article 101792, 2020. paper -
Polynomial splines of non-uniform degree on triangulations: Combinatorial bounds on the dimension.
D. Toshniwal and T.J.R. Hughes.
Computer Aided Geometric Design, 75, Article 101763, 2019. paper, Macaulay2 package -
An isogeometric finite element formulation for phase fields on deforming surfaces.
C. Zimmermann, D. Toshniwal, C.M. Landis, T.J.R. Hughes, K. Mandadapu and R.A. Sauer.
Computer Methods in Applied Mechanics and Engineering, 351, 441-477, 2019. paper -
Blended B-spline construction on unstructured quadrilateral and hexahedral meshes with optimal convergence rates in isogeometric analysis.
X. Wei, Y.J. Zhang, D. Toshniwal, H. Speleers, X. Li, C. Manni, J.A. Evans and T.J.R. Hughes.
Computer Methods in Applied Mechanics and Engineering, 341, 609-639, 2018. paper -
Smooth cubic spline spaces on unstructured quadrilateral meshes with particular emphasis on extraordinary points: Geometric design and isogeometric analysis considerations.
D. Toshniwal, H. Speleers and T.J.R. Hughes.
Computer Methods in Applied Mechanics and Engineering, 327, 411-458, 2017. paper -
Multi-degree smooth polar splines: A framework for geometric modeling and isogeometric analysis.
D. Toshniwal, H. Speleers, R. Hiemstra and T.J.R. Hughes.
Computer Methods in Applied Mechanics and Engineering, 316, 1005-1061, 2017. paper -
High order geometric methods with exact conservation properties.
R. Hiemstra, D. Toshniwal, R.H.M. Huijsmans and M. Gerritsma.
Journal of Computational Physics 257, 1444-1471, 2014. paper -
A geometric approach towards momentum conservation.
D. Toshniwal, R.H.M. Huijsmans and M. Gerritsma.
Spectral and High Order Methods for Partial Differential Equations, Springer, Cham, 393-402, 2014. paper -
The geometric basis of mimetic spectral approximations.
M. Gerritsma, R. Hiemstra, J. Kreeft, A. Palha, P. Rebelo and D. Toshniwal.
Spectral and High Order Methods for Partial Differential Equations, Springer, Cham, 17-35, 2014. paper
Other writings
-
Guest editorial for special issue: Design for Advanced Manufacturing.
Y.F. Zhao, S. Elgeti, J. Guest, C. McComb, J. Norato and D. Toshniwal.
ASME Journal of Mechanical Design, 145, 010301, 2023. editorial -
Interactive design with smooth splines.
D. Toshniwal.
Observable. notebook -
Isogeometric Analysis: Study of non-uniform degree and unstructured splines, and application to phase field modeling of corrosion.
D. Toshniwal.
PhD dissertation, The University of Texas at Austin, USA. thesis